三角函数知识整理思维导图
三角函数知识整理
树图思维导图提供 三角函数知识整理 在线思维导图免费制作,点击“编辑”按钮,可对 三角函数知识整理 进行在线思维导图编辑,本思维导图属于思维导图模板主题,文件编号是:827dc1792779a55f7d739b83c2f7cb63
思维导图大纲
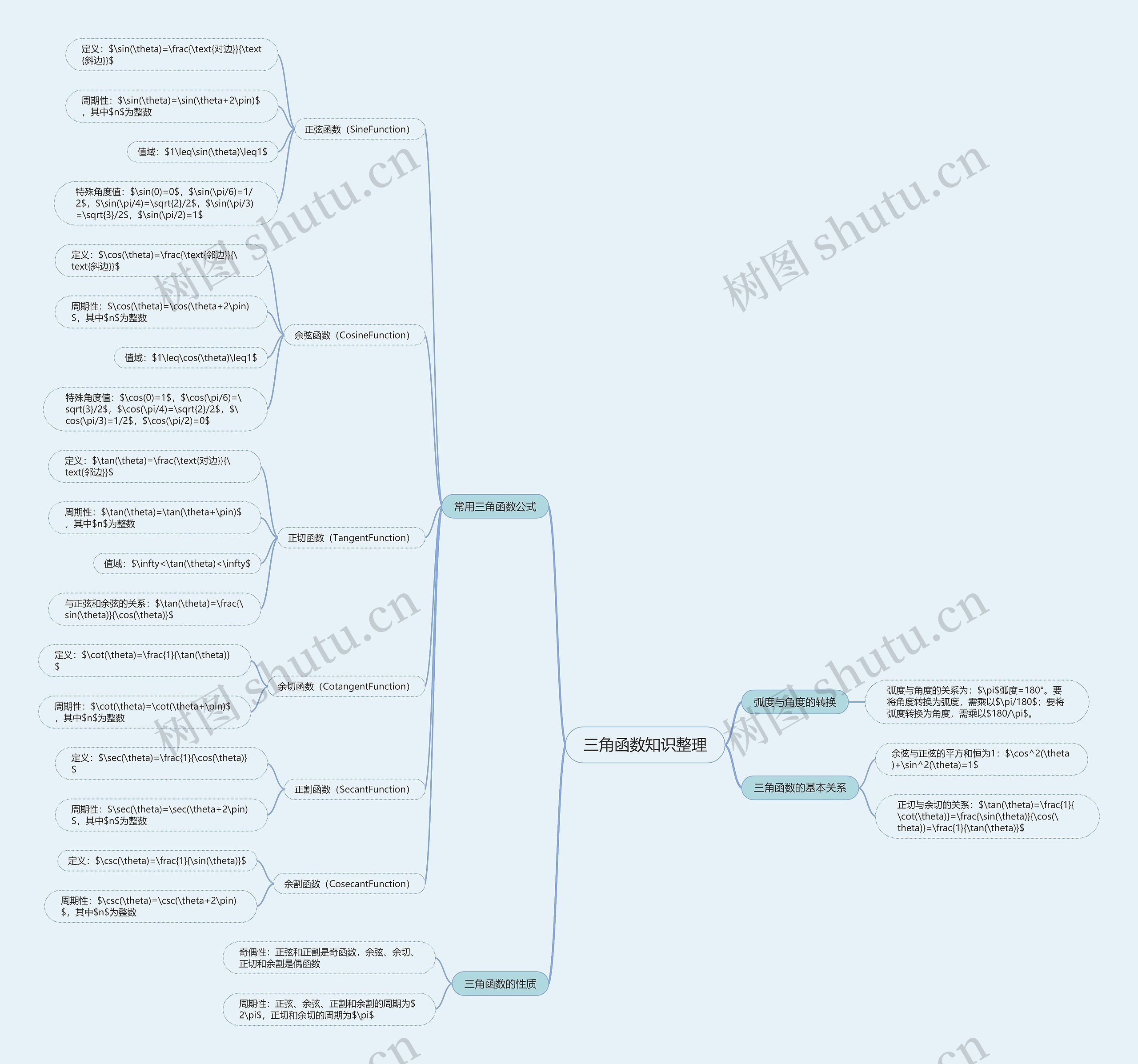
三角函数知识整理思维导图模板大纲
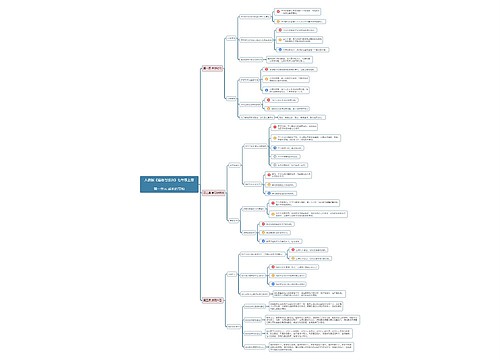
弧度与角度的转换
弧度与角度的关系为:$\pi$弧度=180°。要将角度转换为弧度,需乘以$\pi/180$;要将弧度转换为角度,需乘以$180/\pi$。
常用三角函数公式
正弦函数(SineFunction)
定义:$\sin(\theta)=\frac{\text{对边}}{\text{斜边}}$
周期性:$\sin(\theta)=\sin(\theta+2\pin)$,其中$n$为整数
值域:$1\leq\sin(\theta)\leq1$
特殊角度值:$\sin(0)=0$,$\sin(\pi/6)=1/2$,$\sin(\pi/4)=\sqrt{2}/2$,$\sin(\pi/3)=\sqrt{3}/2$,$\sin(\pi/2)=1$
余弦函数(CosineFunction)
定义:$\cos(\theta)=\frac{\text{邻边}}{\text{斜边}}$
周期性:$\cos(\theta)=\cos(\theta+2\pin)$,其中$n$为整数
值域:$1\leq\cos(\theta)\leq1$
特殊角度值:$\cos(0)=1$,$\cos(\pi/6)=\sqrt{3}/2$,$\cos(\pi/4)=\sqrt{2}/2$,$\cos(\pi/3)=1/2$,$\cos(\pi/2)=0$
正切函数(TangentFunction)
定义:$\tan(\theta)=\frac{\text{对边}}{\text{邻边}}$
周期性:$\tan(\theta)=\tan(\theta+\pin)$,其中$n$为整数
值域:$\infty<\tan(\theta)<\infty$
与正弦和余弦的关系:$\tan(\theta)=\frac{\sin(\theta)}{\cos(\theta)}$
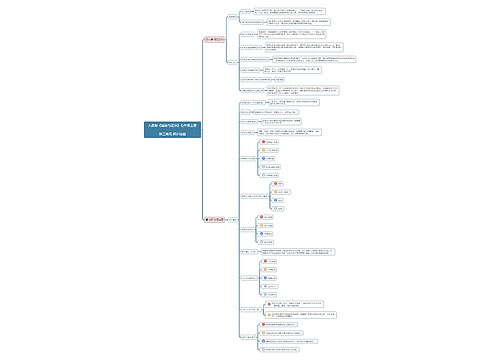
余切函数(CotangentFunction)
定义:$\cot(\theta)=\frac{1}{\tan(\theta)}$
周期性:$\cot(\theta)=\cot(\theta+\pin)$,其中$n$为整数
正割函数(SecantFunction)
定义:$\sec(\theta)=\frac{1}{\cos(\theta)}$
周期性:$\sec(\theta)=\sec(\theta+2\pin)$,其中$n$为整数
余割函数(CosecantFunction)
定义:$\csc(\theta)=\frac{1}{\sin(\theta)}$
周期性:$\csc(\theta)=\csc(\theta+2\pin)$,其中$n$为整数
三角函数的基本关系
余弦与正弦的平方和恒为1:$\cos^2(\theta)+\sin^2(\theta)=1$
正切与余切的关系:$\tan(\theta)=\frac{1}{\cot(\theta)}=\frac{\sin(\theta)}{\cos(\theta)}=\frac{1}{\tan(\theta)}$
三角函数的性质
奇偶性:正弦和正割是奇函数,余弦、余切、正切和余割是偶函数
周期性:正弦、余弦、正割和余割的周期为$2\pi$,正切和余切的周期为$\pi$
相关思维导图模板

树图思维导图提供 2024年8月--2023年8月时政整合 在线思维导图免费制作,点击“编辑”按钮,可对 2024年8月--2023年8月时政整合 进行在线思维导图编辑,本思维导图属于思维导图模板主题,文件编号是:8212d18ea45f416edca4ef534194fc99

树图思维导图提供 科技计划项目资金预算编制总体要求的学习和整理 在线思维导图免费制作,点击“编辑”按钮,可对 科技计划项目资金预算编制总体要求的学习和整理 进行在线思维导图编辑,本思维导图属于思维导图模板主题,文件编号是:49470946de6296e1813ed1ed5efc14ba








 上海工商
上海工商